
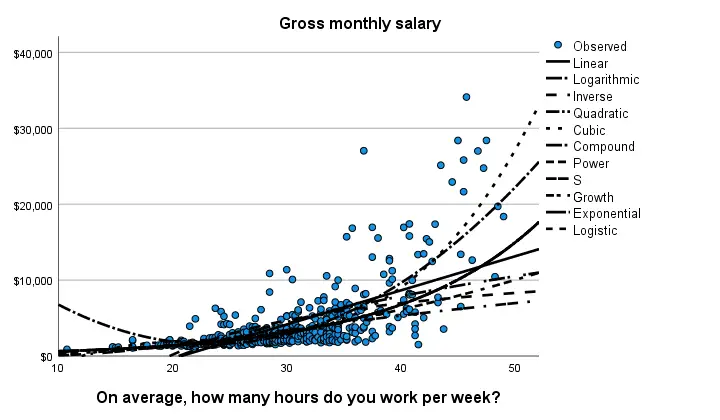
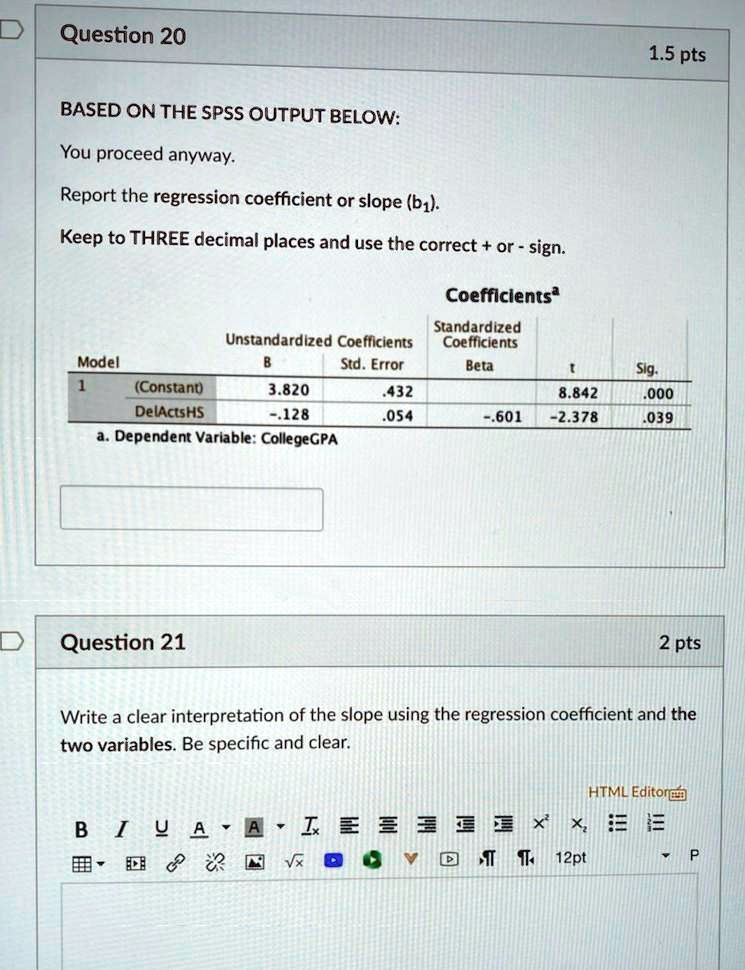
Graphical Presentation House price model: scatter plot Department of Statistics, ITS Surabaya Slide. Sample Data for House Price Model Department of Statistics, ITS Surabaya Slide- House Price in $1000s (Y) Square Feet (X) 245 14 279 17 199 11 405 23 319 14 Simple Linear Regression Example A real estate agent wishes to examine the relationship between the selling price of a home and its size (measured in square feet) A random sample of 10 houses is selected Dependent variable (Y) = house price in $1000s Independent variable (X) = square feet Department of Statistics, ITS Surabaya Slide. Least Squares Method b 0 and b 1 are obtained by finding the values of b 0 and b 1 that minimize the sum of the squared differences between Y and : Department of Statistics, ITS Surabaya Slide-įinding the Least Squares Equation The coefficients b 0 and b 1, and other regression results in this section, will be found using Excel or SPSS Department of Statistics, ITS Surabaya Slide- Formulas are shown in the text for those who are interestedī 0 is the estimated average value of Y when the value of X is zero b 1 is the estimated change in the average value of Y as a result of a one-unit change in X Interpretation of the Slope and the Intercept Department of Statistics, ITS Surabaya Slide. Simple Linear Regression Equation (Prediction Line) Department of Statistics, ITS Surabaya Slide- The simple linear regression equation provides an estimate of the population regression line Estimate of the regression intercept Estimate of the regression slope Estimated (or predicted) Y value for observation i Value of X for observation i The individual random error terms e i have a mean of zero Simple Linear Regression Model Department of Statistics, ITS Surabaya Slide- (continued) Random Error for this X i value Y X Observed Value of Y for X i Predicted Value of Y for X i X i Slope = β 1 Intercept = β 0 ε i Simple Linear Regression Model Department of Statistics, ITS Surabaya Slide- Linear component Population Y intercept Population Slope Coefficient Random Error term Dependent Variable Independent Variable Random Error component Types of Relationships Department of Statistics, ITS Surabaya Slide- Y X Y X No relationship (continued) Types of Relationships Department of Statistics, ITS Surabaya Slide- Y X Y X Y Y X X Strong relationships Weak relationships (continued) Types of Relationships Department of Statistics, ITS Surabaya Slide- Y X Y X Y Y X X Linear relationships Curvilinear relationships Simple Linear Regression Model Only one independent variable, X Relationship between X and Y is described by a linear function Changes in Y are assumed to be caused by changes in X Department of Statistics, ITS Surabaya Slide. Introduction to Regression Analysis Regression analysis is used to: Predict the value of a dependent variable based on the value of at least one independent variable Explain the impact of changes in an independent variable on the dependent variable Dependent variable: the variable we wish to predict or explain Independent variable: the variable used to explain the dependent variable Department of Statistics, ITS Surabaya Slide. Regression A scatter diagram can be used to show the relationship between two variables Correlation analysis is used to measure strength of the association (linear relationship) between two variables Correlation is only concerned with strength of the relationship No causal effect is implied with correlation Department of Statistics, ITS Surabaya Slide.
#LINEAR REGRESSION SPSS 20 HOW TO#
Learning Objectives How to use regression analysis to predict the value of a dependent variable based on an independent variable The meaning of the regression coefficients b 0 and b 1 How to evaluate the assumptions of regression analysis and know what to do if the assumptions are violated To make inferences about the slope and correlation coefficient To estimate mean values and predict individual values Department of Statistics, ITS Surabaya Slide-Ĭorrelation vs. Simple Linear Regression Department of Statistics, ITS Surabaya Slide- Prepared by: Sutikno Department of Statistics Faculty of Mathematics and Natural Sciences Sepuluh Nopember Institute of Technology (ITS) Surabaya


 0 kommentar(er)
0 kommentar(er)
